Lie algebras describe infinitesimal symmetries of physical systems. Therefore, they and their representation theory are extensively used in physics, most notably in quantum mechanics and particle physics. This course introduces semi-simple Lie algebras and the associated Lie groups for physicists. We discuss the essential tools, like the root and weight system, to efficiently work with them and their representations. As on explicit application of the mathematical framework, we discuss Grand Unified Theories (GUT). Moreover, we show how modern computer algebra tools like LieART can significantly help in all explicit computations throughout the course.

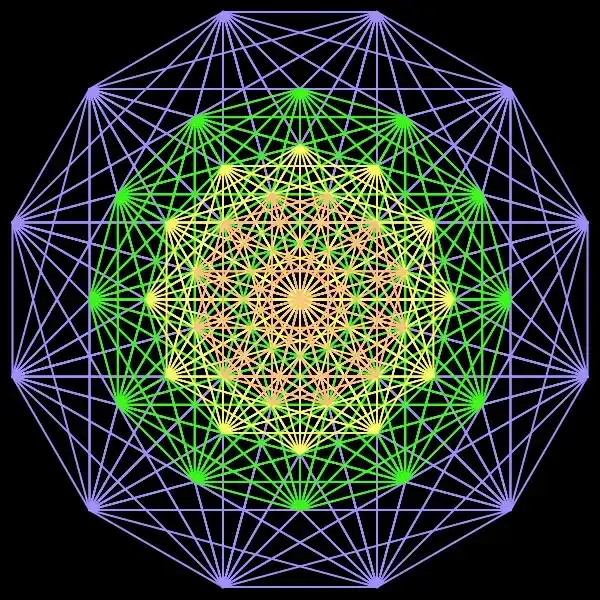A simple example is the visualisation of the root system of $E_6$ projected on the Coxeter plane, which you can see here. If you want to understand how it is created and connected to particle physics, you should take this course. Basic knowledge of core concepts in linear algebra, like vector spaces, eigenvalues and eigenvectors, is assumed. Some good books about the topic are:

• Fuchs and Schweigert: Symmetries, Lie Algebras and Representations
• Gilmore: Lie Groups, Lie Algebras, and Some of Their Applications
• Fulton and Harris: Representation Theory
• Georgi: Lie Algebras In Particle Physics: from Isospin To Unified Theories

The article Phys. Rep. 79 (1981) 1 by Slansky and the manual of the LieART package are good references, too.

Note: At multiple occasions, we will use Mathematica and it might be a good idea to set it up on your computer. Following the instructions on the main teaching website, you should be eventually able to run the notebook, which generates the projection of the $E_6$ root system above.

Exam: After a majority vote, we decided together that the written exam for this course will take place on Tuesday, the 6th of February 2024, at 13:00 in room 447 (the one where we also have our classes). It will take two hours and you have a practise exam to help you preparing for it. Please be there five minutes earlier such that we can start on time.

Retake Exam: As discussed via email, you have the chance to take part in the retake exam on Thursday, the 15th of February 2024, at 13:00 in room 447. Exactly the same conditions as described for the exam above will apply. If you would like to take part in the retake exam, please send me a quick message via email such that I have an estimated head count and can bring the right number of exams.