Lie algebras describe infinitesimal symmetries of physical systems. Therefore, they and their representation theory are extensively used in physics, most notably in quantum mechanics and particle physics. This course introduces semi-simple Lie algebras and the associated Lie groups for physicists. We discuss the essential tools, like the root and weight system, to efficiently work with them and their representations. As on explicit application of the mathematical framework, we discuss Grand Unified Theories (GUT). Moreover, we show how modern computer algebra tools like LieART can significantly help in all explicit computations throughout the course.

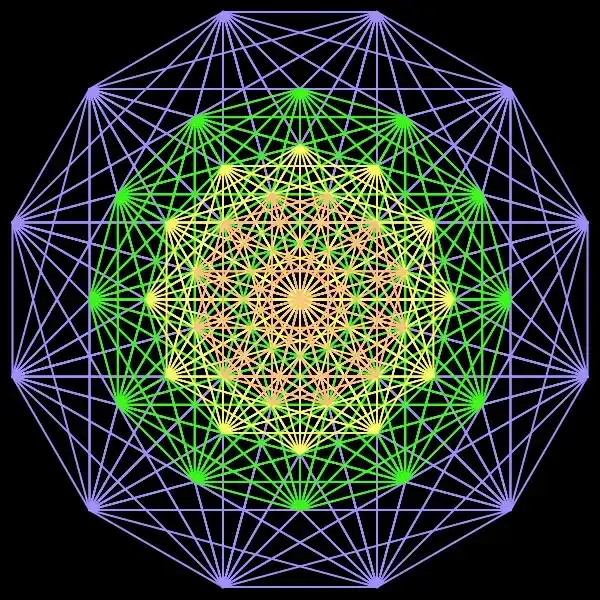A simple example is the visualisation of the root system of $E_6$ projected on the Coxeter plane, which you can see here. If you want to understand how it is created and connected to particle physics, you should take this course. Basic knowledge of core concepts in linear algebra, like vector spaces, eigenvalues and eigenvectors, is assumed. Some good books about the topic are:

• Fuchs and Schweigert: Symmetries, Lie Algebras and Representations
• Gilmore: Lie Groups, Lie Algebras, and Some of Their Applications
• Fulton and Harris: Representation Theory
• Georgi: Lie Algebras In Particle Physics: from Isospin To Unified Theories

The article Phys. Rep. 79 (1981) 1 by Slansky and the manual of the LieART package are good references, too.

Note: At multiple occasions, we will use Mathematica and it might be a good idea to set it up on your computer. Following the instructions below you should be eventually able to run the notebook, which generates the projection of the $E_6$ root system above.

Exam: As we decided together, the exam will be a written exam. It will take place on Friday, the 1st of July 2022 at 10:00 am in room 511. Note that we will start at this time! Please, be there a bit earlier to avoid problems. To prepare for the exam, you should have a look at the practise exam. It may give you a better idea about possible questions and is intended to help you prepare. Take it serious and try to solve it in two hours.

Retake Exam: We now have a consensus for the date of the retake exam. It will take place at Wednesday, the 7th of September 2022 10:00 am in room 447 (the same room where we had the lectures).